ASTM C623-92(1995)e1

NOTICE: This standard has either been superseded and replaced by a new version or discontinued.
Contact ASTM International (www.astm.org) for the latest information.
e1
Designation: C 623 – 92 (Reapproved 1995)
AMERICAN SOCIETY FOR TESTING AND MATERIALS
100 Barr Harbor Dr., West Conshohocken, PA 19428
Reprinted from the Annual Book of ASTM Standards. Copyright ASTM
Standard Test Method for
Young’s Modulus, Shear Modulus, and Poisson’s Ratio for
Glass and Glass-Ceramics by Resonance
This standard is issued under the fixed designation C 623; the number immediately following the designation indicates the year of
original adoption or, in the case of revision, the year of last revision. A number in parentheses indicates the year of last reapproval. A
superscript epsilon (e) indicates an editorial change since the last revision or reapproval.
e NOTE—Section 10 was added editorially in April 1995.
1. Scope possible. A range of acceptable resonance frequencies is
determined for a piece with a particular geometry and density.
1.1 This test method covers the determination of the elastic
Any specimen with a frequency response falling outside this
properties of glass and glass-ceramic materials. Specimens of
frequency range is rejected. The actual modulus of each piece
these materials possess specific mechanical resonance frequen-
need not be determined as long as the limits of the selected
cies which are defined by the elastic moduli, density, and
frequency range are known to include the resonance frequency
geometry of the test specimen. Therefore the elastic properties
that the piece must possess if its geometry and density are
of a material can be computed if the geometry, density, and
within specified tolerances.
mechanical resonance frequencies of a suitable test specimen
1.5 This standard does not purport to address all of the
of that material can be measured. Young’s modulus is deter-
safety concerns, if any, associated with its use. It is the
mined using the resonance frequency in the flexural mode of
responsibility of the user of this standard to establish appro-
vibration. The shear modulus, or modulus of rigidity, is found
priate safety and health practices and determine the applica-
using torsional resonance vibrations. Young’s modulus and
bility of regulatory limitations prior to use.
shear modulus are used to compute Poisson’s ratio, the factor
of lateral contraction.
2. Summary of Test Method
1.2 All glass and glass-ceramic materials that are elastic,
2 2.1 This test method measures the resonance frequencies of
homogeneous, and isotropic may be tested by this test method.
test bars of suitable geometry by exciting them at continuously
The test method is not satisfactory for specimens that have
variable frequencies. Mechanical excitation of the specimen is
cracks or voids that represent inhomogeneities in the material;
provided through use of a transducer that transforms an initial
neither is it satisfactory when these materials cannot be
electrical signal into a mechanical vibration. Another trans-
prepared in a suitable geometry.
ducer senses the resulting mechanical vibrations of the speci-
NOTE 1—Elastic here means that an application of stress within the
men and transforms them into an electrical signal that can be
elastic limit of that material making up the body being stressed will cause
displayed on the screen of an oscilloscope to detect resonance.
an instantaneous and uniform deformation, which will cease upon removal
The reasonance frequencies, the dimensions, and the mass of
of the stress, with the body returning instantly to its original size and shape
the specimen are used to calculate Young’s modulus and the
without an energy loss. Glass and glass-ceramic materials conform to this
shear modulus.
definition well enough that this test is meaningful.
NOTE 2—Isotropic means that the elastic properties are the same in all
3. Significance and Use
directions in the material. Glass is isotropic and glass-ceramics are usually
so on a macroscopic scale, because of random distribution and orientation
3.1 This test system has advantages in certain respects over
of crystallites.
the use of static loading systems in the measurement of glass
1.3 A cryogenic cabinet and high-temperature furnace are
and glass-ceramics:
described for measuring the elastic moduli as a function of
3.1.1 Only minute stresses are applied to the specimen, thus
temperature from −195°C to 1200°C.
minimizing the possibility of fracture.
1.4 Modification of the test for use in quality control is
3.1.2 The period of time during which stress is applied and
removed is of the order of hundreds of microseconds, making
it feasible to perform measurements at temperatures where
This test method is under the jurisdiction of ASTM Committee C-14 on Glass
and Glass Products and is the direct responsibility of Subcommittee C14.04 on
delayed elastic and creep effects proceed on a much-shortened
Physical and Mechanical Properties.
time scale, as in the transformation range of glass, for instance.
Current edition approved Feb. 6, 1992. Published April 1992. Originally
3.2 The test is suitable for detecting whether a material
published as C 623 – 69 T. Last previous edition published edition
meets specifications, if cognizance is given to one important
C 623 – 71 (1989).
Spinner, S., and Tefft, W. E., “A Method for Determining Mechanical
fact: glass and glass-ceramic materials are sensitive to thermal
Resonance Frequencies and for Calculating Elastic Moduli from These Frequen-
history. Therefore the thermal history of a test specimen must
cies,” Proceedings, ASTM, 1961, pp. 1221–1238.
C 623
FIG. 1 Block Diagram of Apparatus
be known before the moduli can be considered in terms of bandwidth before 3-dB power loss occurs.
specified values. Material specifications should include a
4.5 Power Amplifier, in the detector circuit shall be imped-
specific thermal treatment for all test specimens.
ance matched with the type of detector transducer selected and
shall serve as a prescope amplifier.
4. Apparatus
4.6 Cathode-Ray Oscilloscope, shall be any model suitable
4.1 The test apparatus is shown in Fig. 1. It consists of a
for general laboratory work.
variable-frequency audio oscillator, used to generate a sinusoi-
4.7 Frequency Counter, shall be able to measure frequen-
dal voltage, and a power amplifier and suitable transducer to
cies to within 61 Hz.
convert the electrical signal to a mechanical driving vibration.
4.8 If data at elevated temperature are desired, a furnace
A frequency meter monitors the audio oscillator output to
shall be used that is capable of controlled heating and cooling.
provide an accurate frequency determination. A suitable
It shall have a specimen zone 180 mm in length, which will be
suspension-coupling system cradles the test specimen, and
uniform in temperature within 65°C throughout the range of
another transducer acts to detect mechanical resonance in the
temperatures encountered in testing.
specimen and to convert it into an electrical signal which is
4.9 For data at cryogenic temperatures, any chamber shall
passed through an amplifier and displayed on the vertical plates
suffice that shall be capable of controlled heating, frost-free,
of an oscilloscope. If a Lissajous figure is desired, the output of
and uniform in temperature within 65°C over the length of the
the oscillator is also coupled to the horizontal plates of the
specimen at any selected temperature. A suitable cryogenic
oscilloscope. If temperature-dependent data are desired, a
chamber is shown in Fig. 2.
suitable furnace or cryogenic chamber is used. Details of the
4.10 Any method of specimen suspension shall be used that
equipment are as follows:
shall be adequate for the temperatures encountered in testing
4.2 Audio Oscillator, having a continuously variable fre-
and that shall allow the specimen to vibrate without significant
quency output from about 100 Hz to at least 20 kHz. Frequency
restriction. Common cotton thread, silica glass fiber thread,
drift shall not exceed 1 Hz/min for any given setting.
Nichrome, or platinum wire may be used. If metal wire
4.3 Audio Amplifier, having a power output sufficient to
suspension is used in the furnace, coupling characteristics will
ensure that the type of transducer used can excite any specimen
be improved if, outside the temperature zone, the wire is
the mass of which falls within a specified range.
coupled to cotton thread and the thread is coupled to the
4.4 Transducers—Two are required: one used as a driver
transducer. If specimen supports of other than the suspension
may be a speaker of the tweeter type or a magnetic cutting head
type are used, they shall meet the same general specifications.
or other similar device, depending on the type of coupling
chosen for use between the transducer and the specimen. The
other transducer, used as a detector, may be a crystal or
magnetic reluctance type of phonograph cartridge. A capacitive
Smith, R. E., and Hagy, H. E., “A Low Temperature Sonic Resonance
pickup may be used if desired. The frequency response of the
Apparatus for Determining Elastic Properties of Solids,” Internal Report 2195,
transducer shall be as good as possible with at least a 6.5-kHz Corning Glass Works, April, 1961.
C 623
5.3 Specimens shall be finished using a fine grind −400-grit
or smaller. All surfaces shall be flat and opposite surfaces shall
be parallel within 0.02 mm.
6. Procedure
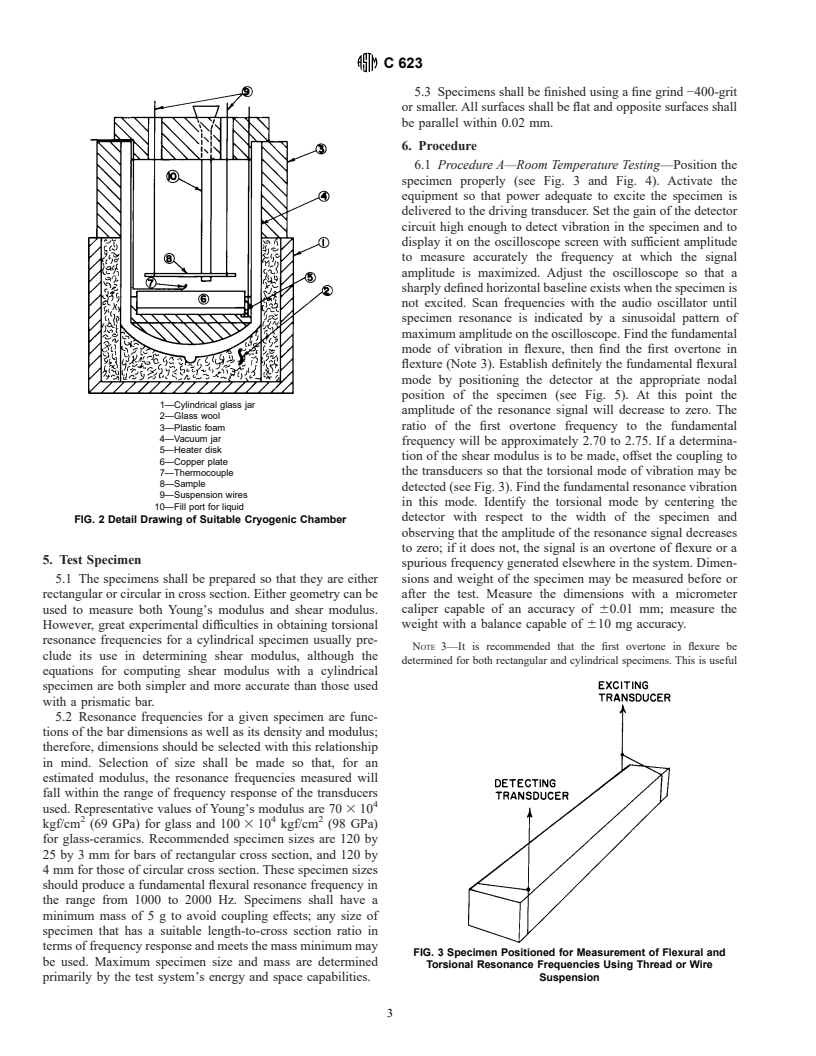
6.1 Procedure A—Room Temperature Testing—Position the
specimen properly (see Fig. 3 and Fig. 4). Activate the
equipment so that power adequate to excite the specimen is
delivered to the driving transducer. Set the gain of the detector
circuit high enough to detect vibration in the specimen and to
display it on the oscilloscope screen with sufficient amplitude
to measure accurately the frequency at which the signal
amplitude is maximized. Adjust the oscilloscope so that a
sharply defined horizontal baseline exists when the specimen is
not excited. Scan frequencies with the audio oscillator until
specimen resonance is indicated by a sinusoidal pattern of
maximum amplitude on the oscilloscope. Find the fundamental
mode of vibration in flexure, then find the first overtone in
flexture (Note 3). Establish definitely the fundamental flexural
mode by positioning the detector at the appropriate nodal
position of the specimen (see Fig. 5). At this point the
1—Cylindrical glass jar
amplitude of the resonance signal will decrease to zero. The
2—Glass wool
3—Plastic foam ratio of the first overtone frequency to the fundamental
4—Vacuum jar
frequency will be approximately 2.70 to 2.75. If a determina-
5—Heater disk
tion of the shear modulus is to be made, offset the coupling to
6—Copper plate
7—Thermocouple the transducers so that the torsional mode of vibration may be
8—Sample
detected (see Fig. 3). Find the fundamental resonance vibration
9—Suspension wires
in this mode. Identify the torsional mode by centering the
10—Fill port for liquid
detector with respect to the width of the specimen and
FIG. 2 Detail Drawing of Suitable Cryogenic Chamber
observing that the amplitude of the resonance signal decreases
to zero; if it does not, the signal is an overtone of flexure or a
5. Test Specimen
spurious frequency generated elsewhere in the system. Dimen-
sions and weight of the specimen may be measured before or
5.1 The specimens shall be prepared so that they are either
rectangular or circular in cross section. Either geometry can be after the test. Measure the dimensions with a micrometer
caliper capable of an accuracy of 60.01 mm; measure the
used to measure both Young’s modulus and shear modulus.
However, great experimental difficulties in obtaining torsional weight with a balance capable of 610 mg accuracy.
resonance frequencies for a cylindrical specimen usually pre-
NOTE 3—It is recommended that the first overtone in flexure be
clude its use in determining shear modulus, although the
determined for both rectangular and cylindrical specimens. This is useful
equations for computing shear modulus with a cylindrical
specimen are both simpler and more accurate than those used
with a prismatic bar.
5.2 Resonance frequencies for a given specimen are func-
tions of the bar dimensions as well as its density and modulus;
therefore, dimensions should be selected with this relationship
in mind. Selection of size shall be made so that, for an
estimated modulus, the resonance frequencies measured will
fall within the range of frequency response of the transducers
used. Representative values of Young’s modulus are 70 3 10
2 4 2
kgf/cm (69 GPa) for glass and 100 3 10 kgf/cm (98 GPa)
for glass-ceramics. Recommended specimen sizes are 120 by
25 by 3 mm for bars of rectangular cross section, and 120 by
4 mm for those of circular cross section. These specimen sizes
should produce a fundamental flexural resonance frequency in
the range from 1000 to 2000 Hz. Specimens shall have a
minimum mass of5gto avoid coupling effects; any size of
specimen that has a suitable length-to-cross section ratio in
terms of frequency response and meets the mass minimum may
FIG. 3 Specimen Positioned for Measurement of Flexural and
be used. Maximum specimen size and mass are determined
Torsional Resonance Frequencies Using Thread or Wire
primarily by the test system’s energy and space capabilities. Suspension
C 623
frequencies to be measured can be detected without further
adjustment. Determine the resonant frequencies at room tem-
perature in the furnace cavity with the furnace doors closed,
etc., as will be the case at elevated temperatures. Heat the
furnace at a controlled rate that does not exceed 150°C/h. Take
data at 25° intervals or at 15-min intervals as dictated by
heating rate and specimen composition. Follow the change in
resonance frequencies with time closely to avoid losing the
identity of each frequency. (The overtone in flexure and the
fundamental in torsion may be difficult to differentiate if not
followed closely; spurious frequencies inherent in the system
may also appear at temperatures above 600°C using certain
types of suspensions, particularly wire.) If desired, data may
also be taken on cooling; it must be remembered, however, that
high temperatures may damage the specimen, by serious
warping for example, making subsequent determinations of
doubtful value.
6.3 Procedure C—Cryogenic Temperature Testing—
Determine the weight, dimensions, and resonance frequencies
FIG. 4 Specimen Positioned for Measurement of Flexural and
in air at room temperature. Measure the resonance frequencies
Torsional Resonance Frequencies Using “Tweeter” Exciter
at room temperature in the cryogenic chamber. Take the
chamber to the minimum temperature desired (Note 4), moni-
toring frequencies as the chamber is cooled. Allow t
...